Xét các số thực x, y thỏa mãn 2^(x^2 + y^2 + 1) < = (x^2 + y^2 - 2x + 2)4^x

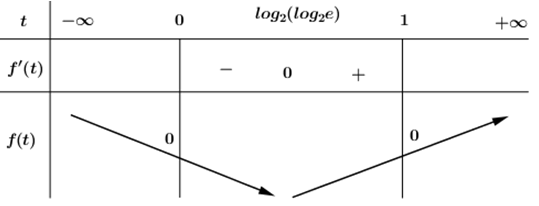
Suy ra ta có \(0 \le t \le 1 \Rightarrow {\left( {x - 1} \right)^2} + {y^2} \le 1\)

Ta có: \(P = \frac{{8x + 4}}{{2x - y + 1}}\)

\[ \Leftrightarrow 2Px - Py + P = 8x + 4\]

\[ \Leftrightarrow P - 4 = \left( {8 - 2P} \right)x + Py\]

\[ \Leftrightarrow 3P - 12 = \left( {8 - 2P} \right)\left( {x - 1} \right) + Py\]

\[ \Leftrightarrow {\left( {3P - 12} \right)^2} \le \left[ {{{\left( {8 - 2P} \right)}^2} + {P^2}} \right]\left[ {{{\left( {x - 1} \right)}^2} + {y^2}} \right]\]

\[ \Rightarrow {\left( {3P - 12} \right)^2} \le {\left( {8 - 2P} \right)^2} + {P^2}\]

\[ \Leftrightarrow 4{P^2} - 40P + 80 \le 0\]

\[ \Leftrightarrow 5 - \sqrt 5 \le P \le 5 + \sqrt 5 \approx 7,23\]

Dấu “=” xảy ra \[ \Leftrightarrow \left\{ {\begin{array}{*{20}{c}}{\frac{{8 - 2P}}{P} = \frac{{x - 1}}{y} = - \frac{2}{{\sqrt 5 }}}\\{{{\left( {x - 1} \right)}^2} + {y^2} = 1}\end{array}} \right. \Leftrightarrow \left\{ {\begin{array}{*{20}{c}}{x - 1 = - \frac{2}{{\sqrt 5 }}y}\\{\frac{9}{5}{y^2} = 1}\end{array}} \right. \Leftrightarrow \left\{ {\begin{array}{*{20}{c}}{x = 1 \mp \frac{2}{3}}\\{y = \pm \frac{{\sqrt 5 }}{3}}\end{array}} \right.\]

\[ \Rightarrow \max P = 5 + \sqrt 5 \] đạt được khi \[x = \frac{1}{3};y = \frac{{\sqrt 5 }}{3}\].