Cho hàm số y = f(x) xác định trên R. Biết rằng hàm số y=f'(x) có đồ thị như hình vẽ Số điểm cực trị của hàm số

* Hướng dẫn giải

Đáp án A.

Set \ (t = {x ^ 2} - 2x \) (with \ (t \ ge - 1), \) phương trình (*) trở thành: \ (f '\ left (t \ right) - \ left ( {t - 1} \ right) = 0 \ Leftrightarrow f '\ left (t \ right) = t - 1 \ left (1 \ right) \)

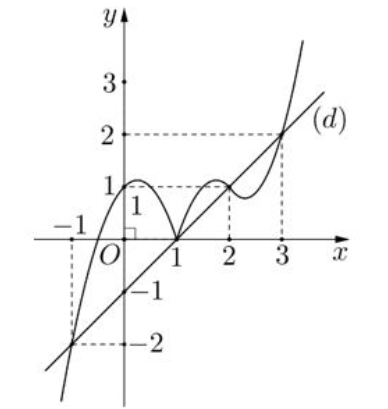
Đồ dùng vào đồ thị hàm số \ (y = f '\ left (x \ right) \) và đồ thị đường thẳng \ (\ left (d \ right): y = x - 1 \)

\ (\ Rightarrow \) File method \ (\ left (1 \ right) \) là \ (\ left \ {{- 1; 1; 2; 3} \ right \} \)

* \ (t = - 1 \ Rightarrow {x ^ 2} - 2x = - 1 \ Leftrightarrow {\ left ({x - 1} \ right) ^ 2} = 0 \ Leftrightarrow x - 1 = 0 \ Leftrightarrow x = 1 \)

* \ (t = 1 \ Rightarrow {x ^ 2} - 2x = 1 \ Leftrightarrow {\ left ({x - 1} \ right) ^ 2} = 2 \ Leftrightarrow x - 1 = \ pm \ sqrt 2 \ Leftrightarrow x = \ pm \ sqrt 2 + 1 \)

* \ (t = 2 \ Rightarrow {x ^ 2} - 2x = 2 \ Leftrightarrow {\ left ({x - 1} \ right) ^ 2} = 3 \ Leftrightarrow x - 1 = \ pm \ sqrt 3 \ Leftrightarrow x = \ pm \ sqrt 3 + 1 \)

* \ (t = 3 \ Rightarrow {x ^ 2} - 2x = 3 \ Leftrightarrow {\ left ({x - 1} \ right) ^ 2} = 4 \ Leftrightarrow x - 1 = \ pm 2 \ Leftrightarrow \ left [ \ begin {array} {l} x = - 1 \\ x = 3 \ end {array} \ right. \)

\ (\ Rightarrow \) Phương trình \ (g '\ left (x \ right) = 0 \) có 6 nghiệm đơn là \ (x = - 1; x = \ pm \ sqrt 2 + 1; x = \ pm \ sqrt 3 + 1; x = 3 \) và có 1 sqrt bội là \ (x = 1. \)

'Number function \ (g \ left (x \ right) = f \ left ({{x ^ 2} - 2x} \ right) - \ left ({\ frac {{{x ^ 4}}} {2} - 2 {x ^ 3} + {x ^ 2} + 2x + 1} \ right) \) có 7 điểm cực trị.