Cho hình chóp S.ABCD có đáy ABCD là hình chữ nhật với

Đáp án B

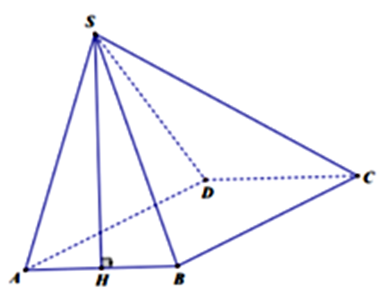
Do \(\left\{ \begin{array}{l}\left( {SAB} \right) \bot \left( {ABC{\rm{D}}} \right)\\\left( {SAB} \right) \cap \left( {ABC{\rm{D}}} \right) = AB\\SH \bot AB\end{array} \right. \Rightarrow SH \bot \left( {ABC{\rm{D}}} \right)\)

Mà \(\Delta SAB\) đều \( \Rightarrow SH = 2{\rm{a}}.\frac{{\sqrt 3 }}{2} = a\sqrt 3 \)

Vậy thể tích hình chóp S.ABCD:

\(V = \frac{1}{3}SH.{S_{ABC{\rm{D}}}} = \frac{1}{3}.a\sqrt 3 .2{\rm{a}}.a\sqrt 2 = \frac{{2\sqrt 6 }}{3}{a^3}\).