Cho hàm số y=f(x) có bảng biến thiên như sau: Số điểm cực tiểu của hàm số

Đáp án C

Ta có: \[g'\left( x \right) = 6{f^2}\left( x \right).f'\left( x \right) + 8f\left( x \right).f'\left( x \right) \Leftrightarrow g'\left( x \right) = 0 \Leftrightarrow \left[ \begin{array}{l}f'\left( x \right) = 0\\f\left( x \right) = 0\\f\left( x \right) = - \frac{4}{3}\end{array} \right..\]

Với \[f'\left( x \right) = 0 \Leftrightarrow \left[ \begin{array}{l}x = - 1\\x = 0\\x = 1\end{array} \right.;\;f\left( x \right) = 0 \Leftrightarrow \left[ \begin{array}{l}x = {x_1} < 1\\x = {x_2} > 1\end{array} \right.;\;f\left( x \right) = - \frac{4}{3} \Leftrightarrow \left[ \begin{array}{l}x = {x_3},{x_3} > {x_1}\\x = {x_4} \in \left( { - 1;0} \right)\\x = {x_5} \in \left( {0;1} \right)\\x = {x_6} > 1,{x_6} < {x_2}\end{array} \right..\]

Vì \[\mathop {\lim }\limits_{x \to + \infty } f\left( x \right) = + \infty \] nên ta có bảng biến thiên cho \[g\left( x \right)\] như sau:

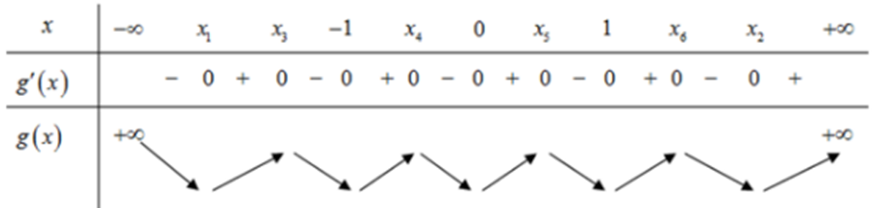
Từ đây ta suy ra số điểm cực tiểu của hàm số \[g\left( x \right)\] là 5.