Bài 4 trang 12 SGK Hình học 10

Đề bài

Cho tam giác \(ABC\). Bên ngoài tam giác vẽ các hình bình hành \(ABIJ, BCPQ, CARS\). Chứng minh rằng \(\overrightarrow{RJ} + \overrightarrow{IQ} + \overrightarrow{PS}=  \overrightarrow{0}.\)

Hướng dẫn giải

Với quy tắc ba điểm tùy ý \(A, \, \, B, \, \, C\) ta luôn có:

\(+ )\;\overrightarrow {AB}  + \overrightarrow {BC}  = \overrightarrow {AC} \) (quy tắc ba điểm).

\( + )\;\overrightarrow {AB}  - \overrightarrow {AC}  = \overrightarrow {CB} \) (quy tắc trừ).

Lời giải chi tiết

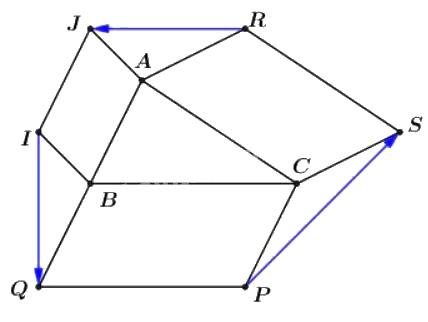
Ta xét tổng:

\(\overrightarrow{RJ} + \overrightarrow{JI} +\overrightarrow{IQ} + \overrightarrow{QP}+\overrightarrow{PS}+ \overrightarrow{SR} \)\(= \overrightarrow{RR}= \overrightarrow{0}\)(1)

Mặt khác, ta có \(ABIJ, BCPQ\) và \(CARS\) là các hình bình hành nên:

\(\overrightarrow{JI}  = \overrightarrow{AB}\)

\(\overrightarrow{QP} = \overrightarrow{BC}\)

\(\overrightarrow{SR}= \overrightarrow{CA}\)

\(\Rightarrow \overrightarrow{JI}+\overrightarrow{QP}+\overrightarrow{SR}\)\(= \overrightarrow{AB}+ \overrightarrow{BC}+\overrightarrow{CA}= \overrightarrow{AA}= \overrightarrow{0}\)  (2)

Từ (1) và (2) suy ra : \(\overrightarrow{RJ} + \overrightarrow{IQ} + \overrightarrow{PS}\)\(=  \overrightarrow{0}.\) (đpcm)