Cho lục giác đều ABCDEF và O là tâm. Có bao nhiêu đẳng thức dưới

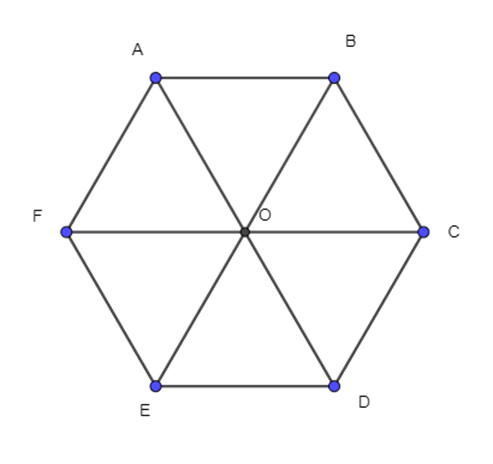
+) Ta có \(\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OE} = \overrightarrow {OA} + \left( {\overrightarrow {OB} + \overrightarrow {OE} } \right) = \overrightarrow {OA} + \overrightarrow 0 = \overrightarrow {OA} \). Do đó A sai.

+) Ta có \(\overrightarrow {BC} + \overrightarrow {FE} = \overrightarrow {AO} + \overrightarrow {OD} = \overrightarrow {AD} \). Do đó B đúng.

+) Ta có \(\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OE} = \overrightarrow {OA} + \left( {\overrightarrow {OB} + \overrightarrow {OE} } \right) = \overrightarrow {OA} + \overrightarrow 0 = \overrightarrow {OA} \ne \overrightarrow {EB} \). Do đó C sai.

+) Ta có \(\overrightarrow {AB} + \overrightarrow {CD} + \overrightarrow {FE} = \overrightarrow {AB} + \overrightarrow {BO} + \overrightarrow {FE} = \overrightarrow {AO} + \overrightarrow {FE} = \overrightarrow {AO} + \overrightarrow {AO} = 2\overrightarrow {AO} \ne \overrightarrow 0 \). Do đó D sai.